In my book Dissections: Plane and Fancy, I presented a prism dissection of two {5/2}-prisms to a {10/4}-prism that uses just 10 compound pieces. The prism dissection is a bit complicated because I use six levels to bridge between the towers, with each of the ten compound pieces having two towers. On the bright side, the dissection is translational, has only three different shapes for the compound pieces, and has identically-cut 5-pointed-star prisms, which are each indistinguishable when turned over from the top to the bottom. Similarly, the 10-pointed star has 2-fold rotational symmetry and is indistinguishable when turned from its top to its bottom. (For the definition of terms like "translational" and "2-fold rotational symmetry," please refer to my book.)
For many years I wanted to produce a physical model of this dissection, with which to illustrate its design and properties. But achieving the necessary precision was daunting. However, the laser-cutting abilities of my friend Walt Hoppe brought this goal within reach. It has taken two tries to be successful, because obtaining precisely-cut flat pieces (108 in all) is only half the battle. The other half is figuring out how to align the flat pieces and keep them in place as the glue dries. My solution was to cut circular holes in the flat pieces of a tower and then insert dowel pins into those holes. If the holes are precisely positioned, then the flat pieces will line up perfectly. Coat the inside of the holes with glue and then insert the dowels. Voilà!
Below is a photo of the ten compound pieces that I glued together from the 108 flat pieces cut by Walt Hoppe with his laser cutter. Each of the two ends of every compound piece contains a tower that is 6 levels high. On each tower you can see the two dowels that lock its 6 flat pieces together and in place. For each of the ten compound pieces, you can see a bridge that links the two towers together. None of the bridges are completely simple, and you may need to study Figures 22.8, 22.9, and 22.10 of Dissections: Plane and Fancy, read the text on pages 263-265 of DPF, and then think about it to understand why the bridges are designed in the way that they are. Note that each piece in the bottom row is the "flip" of the piece above it in the top row. Also note that in the top row, the fifth piece is a flip of the first piece, and the fourth piece is a flip of the second piece. This property also holds for the bottom row, so that altogether there are just three different shapes for the compound pieces!

Next comes the photo of the 10-pointed star prism formed from the ten compound pieces. The perfectly-shaped compound pieces just about leap out at you! Don't you love the 2-fold rotational symmetry?
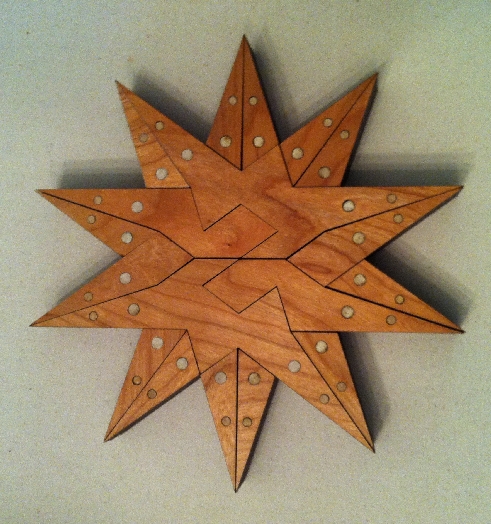
Finally is the photo of the pair of the two 5-pointed star-prisms that can be formed from the ten compound pieces. Enjoy the 2-fold replication symmetry! Too bad that I didn't rotate the stars slightly, to show off the translational property of this dissection! Maybe you can imagine it anyway, with a bit of mental effort!

Even with the remarkable precision available from laser-cutting, it was still a challenge to glue the 108 flat pieces together in a way that allowed me to fit together the resulting ten compound pieces. Only practiced woodworkers should attempt to construct a puzzle like this!
For an update on this project, take a look at my page on adding mother-of-pearl to the cherry wood model of my prism dissection.