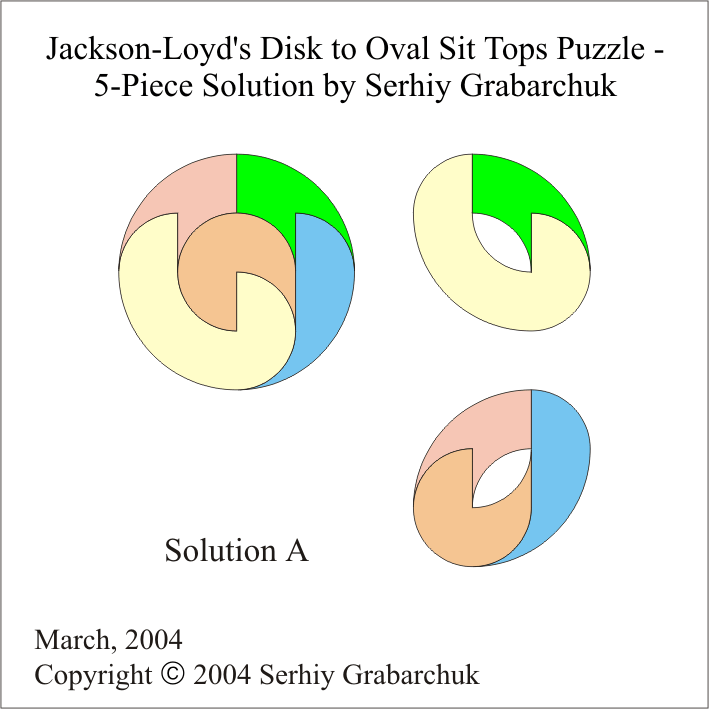
In March 2004, Serhiy Grabarchuk sent me an improvement on Sam Loyd's 6-piece dissection of a disk to two oval seat tops. Serhiy's dissection uses only five pieces, though one is turned over, as we see below.

What a fabulous improvement! So I was wrong in the lead-in to chapter 15 when I wondered if Sam Loyd had brought the puzzle full circle. However, maybe now Serhiy has brought it full circle!
Serhiy sent me a number of different 5-piece dissections of a disk to two oval seat tops. Some of them involved deforming the boundaries between certain pieces. One of them, shown below, had protrusions from one piece to another, by which one cold snap pieces together.
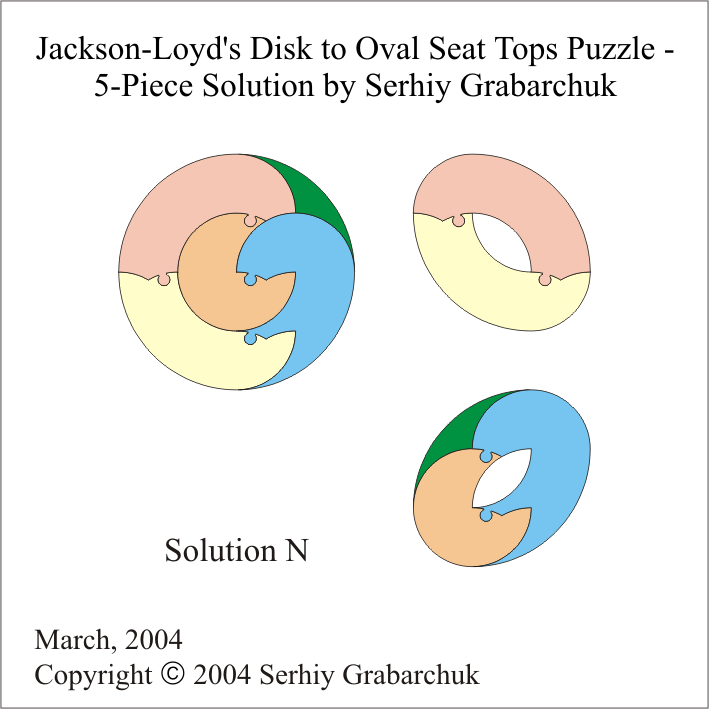
Unfortunately, there is no way to connect the green piece with the others. Then I went and re-examined Serhiy's other solutions and discovered that his solution A could be adapted with snaps. In fact, it is cyclicly snappable: (1) In the disk, blue snaps to brown, which snaps to tan, which snaps to pink, which snaps to green, which snaps to blue. (2) In the top stool, tan snaps to green, which snaps back to tan. (3) In the bottom stool, blue snaps to brown, which snaps to pink, which snaps to blue.
It isn't often that someone comes up with an improved solution for a dissection problem that's been around for almost two centuries, as Serhiy Grabarchuk has done. Thus it seems most appropriate to let everyone know a bit about Serhiy:Serhiy Grabarchuk was born in 1958 in Uzhgorod, Ukraine. He earned a degree in the instruments of fine mechanics from the Kiev Polytechnic Institute, Kiev, Ukraine in 1981. From 1981 to 1989, he worked in several Ukrainian industrial design offices as an engineer-designer, and from 1981 to the present he has been working as a professional puzzle designer and writer.Serhiy creates, designs, collects, solves, makes, produces, and publishes puzzles and puzzle collections. He has created several thousand different puzzles, many of them produced around the world. (See his website Puzzles.com.) He is also captain of the Ukrainian Puzzle Team, and represents Ukraine in the World Puzzle Federation.In addition to puzzles, Serhiy enjoys performing magic and puppet shows, collecting and designing trademarks, music, photography, cinematograph, and different languages.
In August 2007, Gavin Theobald sent me a dissection that is a 1-piece improvement over the Crescent to a Cross that I gave in Figure 15.7. Well done, Gavin!
In approximately 1508-09, Leonardo da Vinci identified a dissection technique for converting an area with matching curved boundaries to an area with rectilinear boundaries. It is essentially the hole technique that Loyd employed in his dissection of a star and a crescent to Crusader's Cross shown in Figure 15.10. In Il Codice Atlantico, Giunti-Barbera, Firenzi, 1973-75, on Foglio 411, Leonardo gave a hole dissection of a crescent to three rectangular areas. Also see Leonardo da Vinci's De Ludo Geometrico, by James Edward McCabe, Ph.D. dissertation, UCLA, 1972, where the figure is discussed and referenced as in the Codex Atlanticus (152 v-a).
In the spring of 1997, Gavin Theobald designed a pretty "globular cross" in which the combined length of the inward curves equals the length of the outward curves. He then found nifty dissections to a square (8 pieces) and to a Greek Cross (10 pieces). Gavin's globular cross is shown on the left below. Each arc has a radius of 2.5 units. His 8-piece dissection to a square is shown on the right, with two pieces turned over.
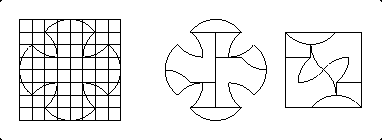
Gavin's cross has all arms the same, like a Greek Cross, so I experimented with a globular cross that is analogous to a Latin Cross, with one arm longer than the rest. I found a 10-piece dissection to a square, and then Gavin found a 10-piece dissection to a Greek Cross. The globular Latin Cross is shown below on the left, and the dissection to a square is on the right. The dissection is based on a tessellation, which you may enjoy finding.

In the March of 2007, Gavin Theobald found a nifty dissection of a globular cross to a square in only seven pieces, with one of the pieces turned over.
Copyright 1997-2007, Greg N. Frederickson.
Permission is granted to any purchaser of
Dissections: Plane & Fancy
to print out a copy
of this page for his or her own personal use.
Last updated August 30, 2007.